Solutions
Get detailed explanations to advanced GMAT questions.
Question
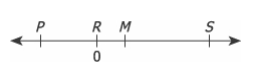
Points P, R, M and S lie on the number line shown. The coordinate of R is 0. The distance between P and R is 1/3 the distance between P and S. If M is the midpoint of line segment PS, what is the coordinate of P?
I. The coordinate of M is 1.5
II. The coordinate of S is 6
Option A:
Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient to answer the question asked.
Option B:
Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient to answer the question asked.
Option C:
BOTH statements (1) and (2) TOGETHER are sufficient to answer the question asked, but NEITHER statement ALONE is sufficient to answer the question asked.
Option D:
EACH statement ALONE is sufficient to answer the question asked.
Option E:
Statements (1) and (2) TOGETHER are NOT sufficient to answer the question asked, and additional data specific to the problem are needed.
Difficulty Level
HardSolution
Option D is the correct answer.
Option Analysis
Let PS = 6x, implying that PR = (1/3)(6x) = 2x and PM = (1/2)(6x) = 3x.
The following number line is yielded:
P<–2x–>R=0<–x–>M<——-3x——->S
In the number line above, P = 0-2x.
Thus, to determine the value of P, we must know the value of x.
Question stem, rephrased: What is the value of x?
Statement I is sufficient: The coordinate of M is 1.5
According to the number line above,
M = 0+x.
Since M=1.5, we get:
1.5 = 0+x
x = 1.5. Sufficient.
Statement II is sufficient: The coordinate of S is 6
According to the number line above,
S = 0 + x + 3x
Since S=6, we get:
6 = 0 + x + 3x
6 = 0 + 4x
x = 1.5. Sufficient
Related Questions
- At the end of each year, the value of a certain antique watch is “c” percent more than its value one…
- The ratio of R to S is 3 to 5, what is the value of the ratio (R + K)/(S + K)?
- Of the 3,600 employees of Company X, 1/3 are clerical. If the clerical staff were to be reduced…
- If 0 < x < 2, which of the following must be greatest?
- To raise funds, a racing team sold T-shirts imprinted with the team’s logo. The team paid their supplier a…
